Evidence of Resonant Waves Found in Rat Brain Activity
Dec 21, 2023
By Dr. Inés Urdaneta, Physicist at the International Space Federation
Using ultrafast and ultrahigh field magnetic resonance imaging (fMRI), researchers at the Champalimaud Foundation and the University of Minho have found evidence of resonant waves in rat brain activity. This means that the brain seems to be a resonant chamber where distant brain areas show correlated activations due to collective wave modes [1].
Many theoretical works have proposed models based on standing waves to explain the macroscopic patterns observed [2-5], though the nature of such activations remains unclear. To delve deeper into this mechanism and understand how distant areas exhibit signal correlations, and how they are implicated in brain function, experimental evidence required a better temporal resolution of the fMRI spectras to show the spatial pattern oscillations and prove the hypothesis that these macroscopic patterns result from the distinct or independent transient oscillation modes at different time scales and wavelengths that add up to generate the resulting overall picture.
This means that the fMRI is reconstructed as the superposition of standing waves, which are a fundamental feature of a resonant cavity. Authors believe that such resonant patters are key for a healthy brain function [6,7]. Standing waves result from constructive interference between waves when subjected to stationary boundary conditions that act as a confinement element for such standing waves to build up.
The video below explains the creation of standing waves in the context of light (electromagnetic waves), which can also be applied to sound waves (mechanical waves that propagate through matter).
To learn more about resonances, please read our RSF article What is Resonance and Why is it so Important?
Mechanical oscillatory motions like sound waves are usually represented by springs that follow a harmonic oscillation, and they obey two main scenarios: ideal (when the oscillations don’t decay in time) or damped (when oscillations decay in time). As we will explain in this article, the authors used the damped case to model the electromagnetic oscillations addressed here.
Intrinsic neural processes have been shown to produce rhythms at frequencies ranging from 0.5 up to >100 Hz, while the role and generative mechanisms of rhythms below 0.5 Hz remain unclear. Using fMRI experiments with unprecedented spatiotemporal resolution, the authors demonstrate the existence of intrinsic macroscale oscillatory modes in fMRI signals which “organize with mode-specific phase relationships across extended areas across the cortex and subcortex, driving correlated activity between distant regions.”[1]. Therefore, the brain geometry should be thought to play a key role as a boundary condition, like a resonant chamber.
In this work researchers experimented with rats in three different conditions: sedated, lightly anesthetized and deeply anesthetized, and they found that the signals in distant brain regions did oscillate together in time, and the spatial configuration of these stationary waves was very consistent across rats scanned in the same condition, but vary in terms of spatial configuration, peak frequency, and damping coefficient across conditions. The modes detected across conditions are qualitatively similar, indicating they likely share a common generative principle.
The visualization of such oscillations was supported on the temporal resolution of 26 images per second, obtaining 16,000 images per 10 minute scan, hence the term ultrafast imaging.
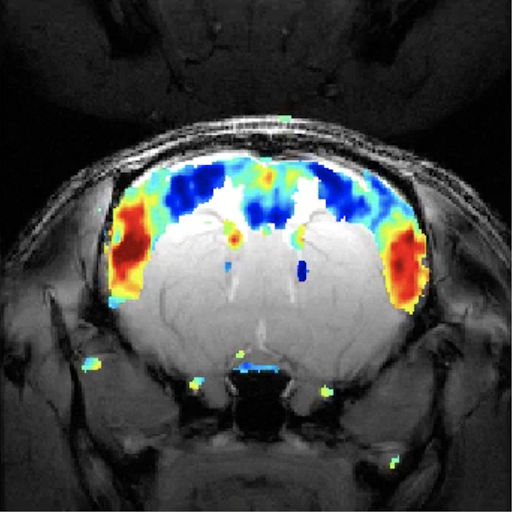Image: captured with fMRI from a rat brain, viewed on top of an anatomical image of the animal. Contralateral areas colored in red activate together at the same time, despite the long distance between them. Credit: Joana Cabral
“Our data show that the complex spatial patterns are a result of transiently and independently oscillating underlying modes, just like individual instruments participate in creating a more complex piece in an orchestra”, - Shemesh, co-athor of the study.
“When we first saw the videos of the recorded brain activity, we saw clear waves of activity, like waves in the ocean, propagating in complex patterns within the cortex and the striatum [a subcortical region of the forebrain]. And we found that the signals could be described by the superposition of a small number of macroscopic stationary waves, or resonant modes, oscillating in time. Notably, each standing wave was found to cover extended areas of the brain, with peaks distributed in distinct cortical and subcortical structures, forming functional networks.” says Cabral, leading author, to Champalimaud Foundation.
Shemesh adds: “We showed that brain functional networks are driven by resonance phenomena. This explains the correlations that are otherwise observed when you do slow imaging. Long-range brain interactions are governed by a ‘flow’ of information that is oscillatory and repetitive.”
Since the main elements detected in rat brain activity are the spatial feature of standing waves and the temporal feature given by the presence of transient oscillations over time, the authors hypothesized that their phenomenology can be associated with the stochastic resonance of standing waves, where the differences detected across conditions are thought to be related to alterations in the properties of the medium through which the waves propagate, while the anatomical structure remains unchanged.
To demonstrate the hypothesis that stochastic resonance of the stationary wave patterns can generate the patterns of intrinsic functional connectivity experimentally observed, the authors modelled the signals in the brain slice as the superposition (linear sum) of modes whose spatial configuration ψα(n) is fixed and given by the principal components detected empirically, while the temporal signature Zα(t) is obtained using an equation known as the Stuart–Landau equation, to simulate the behavior of an oscillator in the underdamped regime and in the presence of background noise.
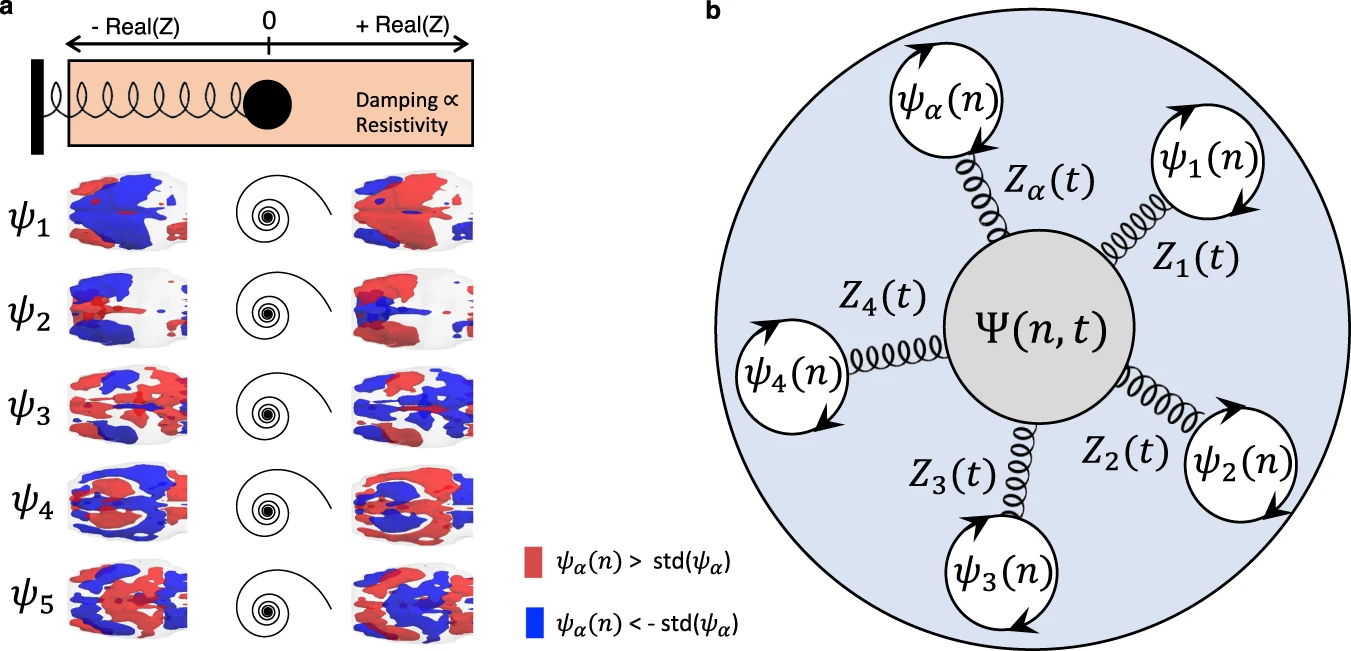Figure 1: a Like the response of spring, the temporal signature of brain modes can be approximated by a damped oscillator. Despite the lower temporal resolution inherent to multi-slice acquisitions hindering the detection of resonant behavior, the consistency of spatial patterns reinforces the hypothesis that the damped oscillatory response of functional networks extends to the whole-brain level, here represented by the first 5 eigenvectors of the average covariance matrices across 6 whole-brain scans (from 3 different rats). b Diagram illustrating a mechanistic scenario for brain activity, where each functional network is represented by a spatial pattern ψαresponding to perturbation with a damped harmonic motion. Figure and text taken from [1].
As shown in Fig. 2 below, the stochastic resonance of a variety of standing waves detected empirically in sedated animals results in a spatiotemporal pattern sharing features with what is detected from fMRI recordings. To complete the whole brain, the process is applied to 12 brain slices.
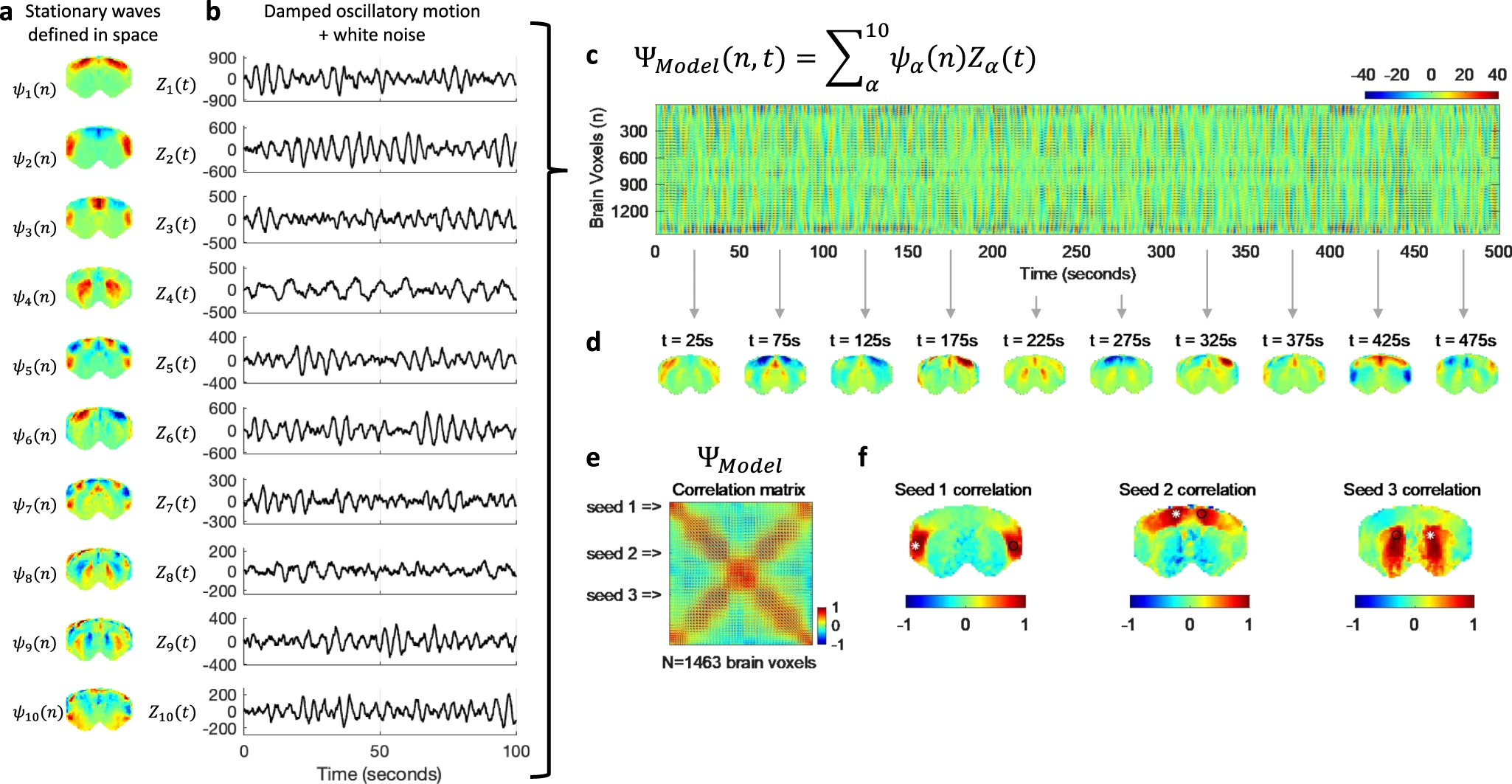Figure 2: The spatial configurations and temporal signatures of the principal components align with the hypothesis that they represent standing waves, whose phenomenology is inherently associated with resonance phenomena. To model the dynamics emerging from the transient resonance of standing waves in the presence of background noise, for each of the spatial patterns detected in medetomidine-sedated rats (a), we simulate a temporal signature as the behavior of an underdamped oscillator perturbed with gaussian white noise (b). c Multiplying each N × 1 spatial pattern by the corresponding 1 × T temporal signature results in an N × T spatiotemporal pattern for each mode. The linear sum of these spatiotemporal patterns represents the superposition of a repertoire of standing waves resonating transiently in the presence of noise. d Still frames of the simulated dynamics obtained at distinct time points reveal the multiplicity of patterns that can be generated over time. e To demonstrate that this scenario generates patterns of long-range functional connectivity, we compute the correlation matrix of the simulated signals, as performed initially on empirical fMRI data (Fig. 1). f The seed correlation maps obtained from the simulated signals reveal correlation patterns visually similar to the ones detected from real fMRI recordings. Figure and caption taken from original work[1].
The authors also found that increasing the amount of anesthetic reduces the number, frequency and duration of the resonant stationary waves. Previous studies have shown that functional networks appear disrupted in several neurological and psychiatric disorders. If this is confirmed in humans, their results could lead to the use of resonant modes as biomarkers for disease.
“Spontaneous fluctuations in signals detected with functional Magnetic Resonance Imaging (fMRI) correlate across spatially distributed brain areas forming functional networks that appear disrupted in numerous psychiatric and neurological disorders, pointing to a key role in brain function”[1].
As Cabral further explaines to Champalimaud Foundation:
“Understanding the mechanism of long-range interactions could lead to a completely new way of characterising disease and hinting on the type of treatment that may be necessary: for example, if a specific resonant mode was missing from a patient, we might want to find ways to stimulate that particular mode.”
These results suggest that the brain works as a whole for certain processes happening within, and this holistic behavior is key for mental health. Their findings also represent a revolutionary approach to design treatments for patients with disordered mental conditions.
Unified Science in perspective –
Resonant processes are one of the main mechanisms through which coherent processes organize from the very small subatomic scale, to the macroscale.
The next paper from Haramein et al. entitled Scale-invariant Unification of Forces, Fields and Particles in a Quantum Vacuum Plasma, shows that the events we call mass (atoms, cells, planets, stars, solar systems, galaxies) are in a clear radii-to-mass relationship. This suggests that the universe itself can be understood as a resonant chamber where a resonant condition organizes in a coherent manner these events that go all the way from the Planck scale, up to the universal scale, like the harmonics of a musical piece producing a fractal-holographic melody …
References
[1] Cabral, J., Fernandes, F.F. & Shemesh, N. Intrinsic macroscale oscillatory modes driving long range functional connectivity in female rat brains detected by ultrafast fMRI. Nat Commun 14, 375 (2023). https://doi.org/10.1038/s41467-023-36025-x
[2] Breakspear, M. Dynamic models of large-scale brain activity. Nat. Neurosci. 20, 340–352 (2017).
[3] Henderson, J. A., Aquino, K. M. & Robinson, P. Empirical estimation of the eigenmodes of macroscale cortical dynamics: reconciling neural field eigenmodes and resting-state networks. Neuroimage 2, 100103 (2022).
[4] Jirsa, V. K. & Haken, H. Field theory of electromagnetic brain activity. Phys. Rev. Lett. 77, 960 (1996).
[5] Gabay, N. C. & Robinson, P. Cortical geometry as a determinant of brain activity eigenmodes: Neural field analysis. Phys. Rev. E 96, 032413 (2017).
[6] Belloy, M. E. et al. Quasi-periodic patterns of neural activity improve classification of Alzheimer’s disease in mice. Sci. Rep. 8, 1–15 (2018).
[7] van den Berg, M. et al. Altered basal forebrain function during whole-brain network activity at pre-and early-plaque stages of Alzheimer’s disease in TgF344-AD rats. Alzheimer’s Res. Ther. 14, 1–21 (2022).
[8] Nassim Haramein & Olivier Alirol. Scale-invariant Unification of Forces, Fields and Particles in a Quantum Vacuum Plasma.
