"Missing Law" Proposed that Describes a Universal Mechanism of Selection for Increasing Functionality in Evolving Systems

By: William Brown, biophysicist at the International Space Federation

• A recently released research article has proposed a “law of increasing functional information” with the aim of codifying the universally observed behavior of naturally evolving systems—from stars and planets to biological organisms—to increase in functional complexity over time.
• To codify this behavior, it is proposed that functional information of a system will increase (i.e., the system will evolve) if many different configurations of the system undergo selection for one or more functions.
• Note that “evolution” is being used in a general sense, as Darwinian evolution is regarded as specific to the biological system and requires heritable material or some form of transmissible and stable memory from one iterative variant to the next, which is conventionally not considered as operable in generic dynamic physical systems, although theories like the Unified Spacememory Network and Morphic Resonance can extend the special case of Darwinian evolution to dynamic physical systems in general as they posit a medium of transmissible memory via spacememory or a morphogenic field, respectively.
• An “evolving system” is defined as a collective phenomenon of many interacting components that displays a temporal increase in diversity, distribution, and patterned behavior. As such, evolving systems are a pervasive aspect of the natural world, occurring in numerous natural contexts at many spatial and temporal scales.

While not the first such study to propose a universal mechanism to explain the near-ubiquitous observable tendency of diverse natural systems to evolve to ever increasing levels of complexity—and outstandingly, functional complexity at that—a rigorous codification approaching the level of a “natural law”—like the laws of motion, gravity, or thermodynamics—is significant. In our study The Unified Spacememory Network we proposed just such a law that generic natural systems will evolve to ever increasing levels of synergetic organization and functional complexity. In the Unified Spacememory Network approach, the ever-increasing levels of functional information of naturally evolving systems is in-part a function of the memory properties of space. In the recent study, researchers utilize a comparative analysis of equivalencies among naturally evolving systems—including but not limited to life—to identify further characteristics of this “missing law” of increasing functional complexity such as the observation that all evolving systems are composed of diverse components that can combine into configurational states that are then selected for or against based on function, and as the (often very large) configurational phase space is explored those combinations that are maximally functional will be selected for preferentially. The study also proposes mechanisms subsumed within the law of increasing functional information that account for the tendency of evolving systems to increase in diversity and generate novelty.

Universality of Evolving Systems

So certain is this that we may boldly state that it is absurd for human beings to even attempt it, or to hope that perhaps some day another Newton might arise who would explain to us, in terms of natural laws unordered by intention, how even a mere blade of grass is produced. Kant, Critique of Judgement (1790)

The universe is replete with complex evolving systems—the universe itself can be considered an evolving system (Figure 1)—and a major endeavor of unified science is to understand and codify the underlying dynamics generating evolving systems and resulting complexification, whether spontaneous emergence in self-organizational systems or delineable underlying ordering mechanisms that verge on operational “laws of nature”. From studies such as A unifying concept for Astrobiology by E.J. Chaisson that quantitatively defines evolving systems based on energy flow, such that all ordered systems—from rocky planets and shining stars, to buzzing bees and redwood trees—can be judged empirically and uniformly by gauging the amount of energy acquired, stored and expressed by those systems [1], to biophysicist Antonis Mistriotis’ universal model describing the structure and functions of living systems [2] in which evolving systems like life are identified as “far-from-the-equilibrium thermodynamic phenomenon that involves the creation of order (reduction of internal entropy) by accumulating and processing information,” there is a strong foundation within this field of investigation for understanding the physics of life and complex evolving systems in general.  

An open question within understanding the complexification of matter over time is whether there are natural laws—akin to the codification of statistical averages as laws underlying thermodynamics—that are operational in generic complex dynamical systems that can be characterized as having an asymmetric-time evolution. Chaisson defines complexity as: “a state of intricacy, complication, variety or involvement, as in the interconnected parts of a system—a quality of having many interacting, different components” and notes that “particularly intriguing is the potentially dramatic rise of complexity within the past half-billion years since the end of the pre-Cambrian on Earth. Perhaps indeed resembling a modern form of Platonism, some underlying principle, a unifying law, or an ongoing process creates orders and maintains all structures in the Universe, enabling us to study all such systems on a uniform, level ground" [1, pg.93]. 

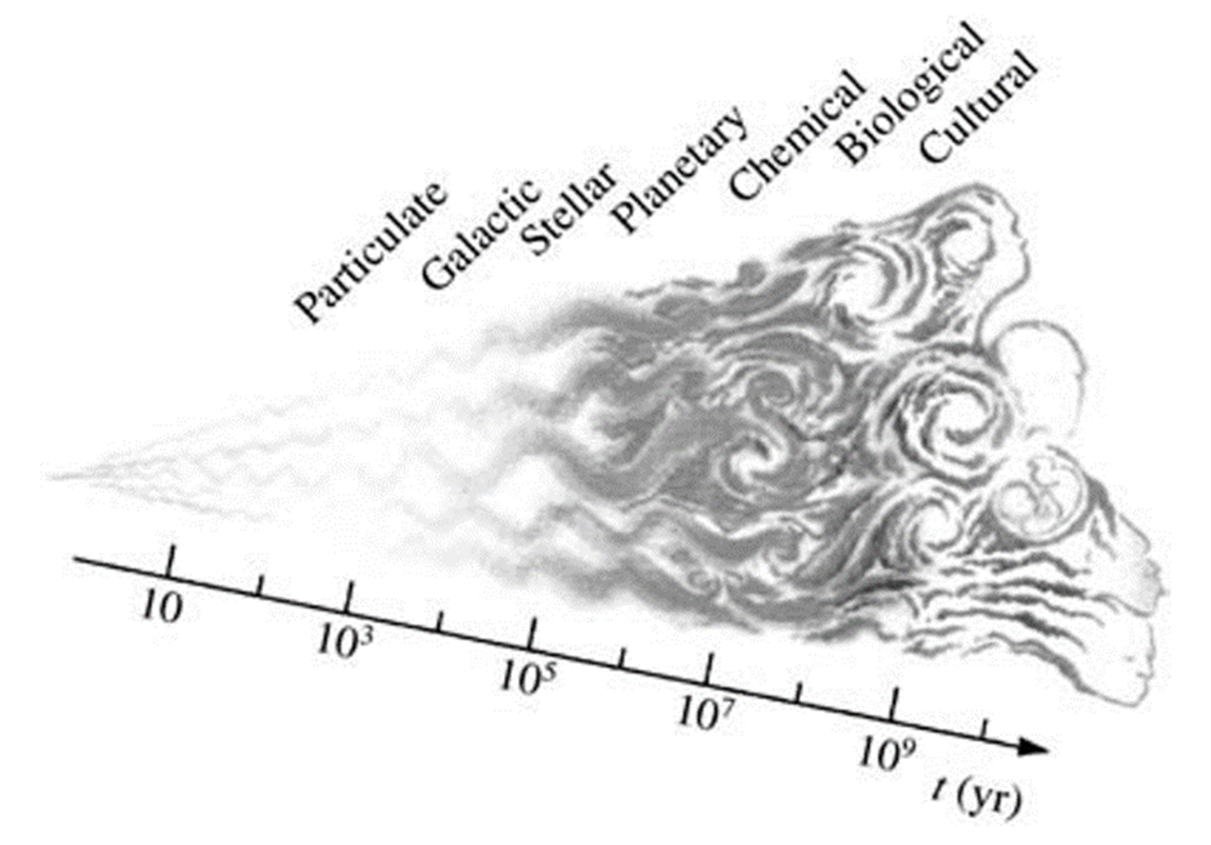
_{Figure 1. A stylized arrow of time highlighting the salient features of cosmic history in terms of an evolutionary process. From its supposed high-energy origins some 14 GA (left) to the here and now of the present (right) with complex evolving systems giving rise to culture, cybernetics, and AI. Labelled diagonally across the top are the major evolutionary phases that have produced, in turn, increasing amounts of order and complexity among all material systems: particulate, galactic, stellar, planetary, chemical, biological, and cultural evolution. Cosmic evolution encompasses all of these phases. Image and image description from Chaisson [1].}

Now, there has been new advancement in this investigation into the nature of complex evolving systems as a recently release study On the Roles of Function and Selection in Evolving Systems, by Wong et al., in the journal PNAS [3], discusses how existing macroscopic physical laws (Table 1) do not seem to adequately describe these dynamical systems found all throughout nature and that there must be a veritable "missing law" that is operable in driving the asymmetric increase in functional complexity of complex evolving systems over time.

Historically it has been generally accepted that there is no such equivalent universal law operational in the development and evolution of dynamic systems describing a tendency to increase in functional complexity because it is assumed that the underlying dynamics are intrinsically stochastic (randomly determined; having a random probability distribution or pattern that may be analyzed statistically but may not be predicted precisely) and therefore any general developmental or complexification process proceeds via one random accident after another with no underlying natural directionality, ordering process, or mechanism that would equate to a physical law from which, for example, a near-precise probability outcome could be calculated for the behavior and trajectory of any given evolving system, to the limit that certain complex dynamic systems are time-symmetric and non-deterministic (see for example the Belousov–Zhabotinsky reaction). For complex evolving systems of the biological variety, natural selection can act on permutations when they arise and is an effective ordering mechanism in that way, but it cannot generate the novelty and changes on which it acts; in orthodox theory that is left to random chance. 

As such, in studies like The Astrobiological Copernican Weak and Strong Limits for Intelligent Life [4], by Westby and Conselice, where utilizing data to calculate the prevalence of intelligent life in the Milky Way galaxy the researchers must resort to a probabilistic analysis that takes into account a range of possibilities from a “strong scenario” with strict assumptions on the improbability of matter evolving into living organisms on any given habitable exoplanet to an “ultraweak scenario” that is more permissive in the underlying assumptions [Table 2]. So, for example, under the most permissive (ultraweak) assumptions they calculate a prevalence of approximately 4.63 X 10¹⁰ (~40 billion) number of occurrences of primitive life developing on planets in our galaxy, while under the most stringent (strong) assumptions they find that there should be at least 36 intelligent (communicating) civilizations in our galaxy, if however restrictions are loosened and calculations are made under the assumption that life has a relatively decent probability of developing on rocky planets where there is liquid water and a consistent low-entropy energy source then the number of potential intelligent civilizations in our galaxy is exponentially larger than a mere 36.

If there were known macrophysical laws delineating the behavior of evolving systems, the researchers Wesby and Conselice would not have to rely on “assumptions” for their analysis. Aside from the seemingly unscientific capitulation of attributing development of generic evolving systems—not just life—to randomness that is prevalent within conventional academia, or relying on purely emergent ordering behavior that can be spontaneously exhibited in self-organizing systems [see Kauffman, 5], this orthodox purview seems to neglect significant observables like the uniform increase in complexity and diversity of matter that is readily evident over the universe’s history and the remarkable instance of matter to organize into the superlative functionally complex system of the living organism. Indeed, the predominance of the assumption that randomness is fundamental, and emergence of order is most rationally attributed only to coincidental or serendipitous accidents leads theorists to posit that it must be extremely unlikely for something like biogenesis to occur, despite the more general observation that material systems across space and time have an inexorable tendency towards complexification and functional synergetic organization.

For example, Andrew Watson in Implications of an Anthropic Model of Evolution for Emergence of Complex Life and Intelligence [6] deduces that there is only a 2.6% chance for one of the major transitions in evolution of primordial molecular replicating systems to become living cells (Table 3), suggesting that it is highly unlikely for circumstances to permit biogenesis within timeframes similar to the known lifespan of Earth’s biosphere (supporting the “rare Earth” hypothesis). However, if we take into consideration the infodynamics operable from a guiding field, like Meijer et al’s informational quantum code [7], Sheldrake’s Morphic Resonance [8], or Chris Jeynes and Michael Parker’s Holomorphic Info-Entropy concept [9], we can make a rational inference that organizational dynamics, verging on a veritable effective entropic force or physical law of complexity, will drive systems to ever increasing functional organization and biogenesis will be a relatively likely and universal outcome wherever conditions are favorable for biological habitability.

This assumption within the orthodox approach is, however, shifting even within conventional circles. Evaluating the uniform increase in complexity and functionality of physical systems in the universe, Wong et al. have derived a physical law that they propound underlies the behavior of evolving systems, in which the functional information of a system will increase (i.e., the system will evolve) if many different configurations of the system undergo selection for one or more functions. By identification of conceptual equivalencies among disparate phenomena—a process that was foundational in developing previous laws of nature like those in Table 1—the research team purports to identify a potential “missing law”. They postulate that evolving systems—including but not limited to the living organism—are composed of diverse components that can combine into configurational states that are then selected for or against based on function. Hence, via a delineation of the fundamental sources of selection: (1) static selection, (2) dynamic persistence, and (3) novelty generation; Wong et al. have proposed a time-asymmetric law that states that the functional information of a system will increase over time when subjected to selection for function(s).

The Law of Increasing Functional Information

The laws presented in Table 1 are some of the most important statements about the fundamental behavior of physical systems that scientists have discovered and articulated to date, yet as Wong et al. point out in their recent study, one conspicuously absent statement is a law of increasing complexity. Nature is replete with examples of complex systems and a pervasive wonder of the natural world is the evolution of varied systems, including stars, minerals, atmospheres, and life (Figure 2). The study On the Roles of Function and Selection in Evolving Systems delineates at least 3 definitive attributes of evolving systems that appear to be conceptually equivalent:  1) they form from numerous components that have the potential to adopt combinatorially vast numbers of different configurations; 2) processes exist that generate numerous different configurations; and 3) configurations are preferentially selected based on function. Universal mechanisms of selection and novelty generation—outlined below—drive such systems to evolve via the exchange of information with the environment and hence the functional information and complexity of a system will increase if many different configurations of the system undergo selection for one or more functions.

_{Figure 2. The history of nature from the Big Bang to the present day shown graphically in a spiral with notable events annotated. Every billion years (Ga) is represented by a 90-degree angle section of the spiral. The last 500 million years are represented in a 90-degree stretch for more detail on our recent history. Some of the events depicted are the emergence of cosmic structures (stars, galaxies, planets, clusters, and other structures), the emergence of the solar system, the Earth and the Moon, important geological events (gases in the atmosphere, great orogenies, glacial periods, etc.) and the emergence and evolution of living beings (first microbes, plants, fungi, animals, hominid species)}

Origin of Selection and Function

Lets now take a closer look at the 3 attributes identified and delineated by Wong et al. in their study; the three definitive attributes of evolving systems being:  (1) static selection, identified as the principle of static persistence; (2) dynamic persistence, identified as the principle of the persistence of processes; and (3) novelty generation, a principle of selection for novelty.

Principle of static persistence (first-order selection)- configurations of matter tend to persist unless kinetically favorable avenues exist for their incorporation into more stable configurations. As described by Wong et al. persistence provides not only an enormous diversity of components but “it also provides ‘batteries of free energy’ or ‘pockets of negentropy’ throughout the universe that fuel dynamically persistent entities”.

The research team derived the first-order selection parameter of the law of increasing complexity by imagining an alternate universe that begins like our own but ultimately does not produce any systems of increasing complexity. As described, “in such a patternless world, systems smoothly march toward states of higher entropy without generating any long-lived pockets of low entropy or ‘pockets of negentropy’, for example, because of an absence of attractive forces (gravity, electrostatics)” or constants like alpha are not “fine-tuned” and stable atoms cannot even form. In our study The Unified Spacememory Netwok [10], we followed a similar thought-experiment to illustrate the mechanism of increasing synergic organization and functional complexity via the memory attribute of the multiply-connected spacetime manifold and neuromorphic ER=EPR-like connectivity architecture of the Spacememory Network (Figure 3).

_{Figure 3. (A) Potential paths of the evolution of matter in the Universe (for conceptual illustration only). Arrows indicate the relative degree of probability under conventional models, with potential path 1 having the strongest degree of probability, but the lowest degree of order and complexity; potential path 2 having the lowest degree of probability, but the highest degree of ordering and complexity; and potential path 3 having a median probabilistic expectation value. (B) Postulated effect of nonlocal interactions (EPR correlations) of the ERb=EPR micro-wormhole information network on the development and evolution of atomic and molecular structures in the universe. The high density ERb=EPR micro-wormhole connections integral to complex and highly ordered molecules (pathway 2) produce a stronger interaction across the temporal dimension, as well as intramolecularly. This influences the interactivity of atoms such that there is a veritable force driving the systems to form complex associations – a negentropic effect. The trans-temporal information exchange, that appears as a memory attribute of space, is an ordering effect that drives matter in the universe to higher levels of synergistic organization and functional complexity.}

Similar to our conclusion in The Unified Spacmemory Network, Wong et al. conclude that states of matter in our universe do not march smoothly to maximal entropy (pathway 1 in Figure 3), but instead there are negentropic forces that “frustrates” the dissipation of free energy “permitting the long-lived existence of disequilibria” and resulting in “pockets of negentropy” that fuel dynamically persistent entities (pathway 2 in Figure 3).

The significance of the property of certain states of matter to decrease entropy, maintain, and perpetuate far-from-equilibrium thermodynamic states as part of the complexification of evolving systems, leading to the living organism, has been pointed in previous studies like the author’s work on defining the key transition from abiotic to biotic organized matter [11]. Mistriotis has further elucidated the mechanism of negentropic action by the living systems as involving the processing of information, whereby via logic operations, like that of an electronic circuit, the internal entropy of a complex evolving system like the living organism is decreased and hence, all living systems necessarily perform logical operations similar to electronic circuits. Logic is necessary in the living system to perform the self-similar functions of decreasing entropy across the hierarchical organization of the organism, such that the similarity with the information processing of an electronic circuit is elaborated even further to draw similarities with the read-write functionality of computer memory, showing that complex evolving systems like life are processing information at a complex level [12].

Second-order selection, persistence of processes- this second postulate defines the characterization of “function” that may be attributed to a process and how functionality is ultimately selected for as opposed to process that do not contribute to the causal efficacy over internal states of a system. As described by Wong et al. “insofar as processes have causal efficacy over the internal state of a system or its external environment, they can be referred to as functions. If a function promotes the system’s persistence, it will be selected for.”

Third-order selection for novelty- the third-order selection parameter addresses a significant challenge in evolutionary theory, in which natural selection can describe the selection and preservation of adaptive phenotypes but cannot explain the de novo generation of novelty [13]. This is addressed in the new study by positing that “there exist pressures favoring systems that can open-endedly invent new functions—i.e., selection pressures for novelty generation.” Adding new functions that promote the persistence of the core functions essentially raises a dynamic system’s “kinetic barrier” against decay toward equilibrium. The new study further elaborates: “a system that can explore new portions of phase space may be able to access new sources of free energy that will help maintain the system out of equilibrium or move it even further from equilibrium. In general, in a universe that supports a vast possibility space of combinatorial richness, the discovery of new functional configurations is selected for when there are considerable numbers of functional configurations that have not yet been subjected to selection.”

Like Mike Levin’s scale-free cognition and complex organization of compound intelligences [14], in a more general sense Wong et al. point out the most complicated systems are nested networks of smaller complex systems, each persisting and helping to maintain the persistence of the whole. Importantly, in nested complex systems, ancillary functions may arise, like eddies swirling off a primary flow field.

The first, second, and third order selection-for-function parameters are proposed to account for the origins of selection and function, since the universe that we observe constantly generates certain ordered structures and patterned systems whose existence and change over time cannot adequately be explained by the hitherto identified laws of nature, such as those summarized in Table 1. These postulates lead to the formalization of a kind of law to describe the increase in system complexity through the existence of selection pressures:

Systems of many interacting agents display an increase in diversity, distribution, and /or patterned behavior when numerous configurations of the system are subject to selective pressures.

As such, there is a universal basis for selection and a quantitative formalism rooted in the transfer of information between an evolving system and its environment.

Functional Information and the Evolution of Systems

All of the natural laws in Table 1 involve a quantitative parameter such as mass, energy, force, or acceleration, and it naturally moots the question, “is there an equivalent parameter associated with evolving systems?” The latest study expounds that indeed there is, and the answer is information (measured in bits), specifically “functional information” as introduced in studies like Functional Information and the Emergence of Biocomplexity [15]. Functional information quantifies the state of a system that can adopt numerous different configurations in terms of the information necessary to achieve a specified “degree of function,” where “function” may be as general as stability relative to other states or as specific as the efficiency of a particular enzymatic reaction.

In the hierarchy of increasing complexity that characterizes the biological system, Mistriotis identifies the characteristic of Functional self-similarity, where like a fractal that repeats an elementary pattern with fixed geometric characteristics recursively to generate a complex self-similar structure, the nested hierarchical levels of the living organisms repeat functions like metabolism, growth, reproduction, and responsiveness in a self-similar pattern across each organizational domain. As such, “as fractal geometry requires an elementary pattern that acts as a seed, Functional Self-similarity implies the existence of an elementary living system” [16].

Regarding the law of increasing functional information for generic evolving systems, the functional information formalism points to an important universal characteristic:

The functional information of a system will increase (i.e., the system will evolve) if many different configurations of the system are subjected to selection for one or more functions.

As described by Wong et al. this postulate is a close parallel to the previously proposed law of increasing complexity, which states that natural selection, acting alone, tends to increase the complexity of a system [17].  It is interesting to consider how this relates mechanistically to holographic physics, whereby an increase in information and, according to this study, the corresponding system complexification will be correlated with increasing spacetime hypersurfaces, as information is encoded on spacetime surface area as spacememory. The connnectivity circuits of these spacetime hypersurfaces, known as Einstein-Rosen bridges, may explain mechanistically the increasing functional complexity, aside from selection pressures only, and the corresponding increase in synergistic organization of complex functional systems. 

Moving Towards Codifying Mechanisms of Complexification and Function as Natural Laws

The tendency for diversity and complexity to increase in physical systems has been discussed in detail in previous works, such as the proposal of Biology’s First Law by McShea and Brandon, in which they postulate a zero-force evolutionary law that states that:

In any evolutionary system in which there is variation and heredity, there is variation and heredity, there is a tendency for diversity and complexity to increase, one that is always present but may be opposed or augmented by natural selection, other forces, or constraints acting on diversity or complexity [18].

While McShea and Brandon’s proposal for a zero-force evolutionary law applies exclusively to evolutionary systems in which there is variation and heredity, and therefore is narrowly specified to living organisms (that have the hereditary capacity bestowed by the chemical memory of the DNA-RNA-protein system), the work of Wong et al expands this to generic physical systems, and in our Unified Spacememory Network postulate we describe the mechanism by which generic physical systems, or organized matter in the universe, obey a universal evolutionary force—including ever-increasing functional information, synergetic organization, complexity and diversity—via the memory properties of space.

Even within the domain of purely theoretical physics, in analysis of complexity theory, there has been proposals for concepts such as The Second Law of Quantum Complexity, by Susskind and Brown [19], where it is shown that long after a system reaches maximal entropy, its evolution is not over as at a quantum level it continues to explore combinatorial phase-space and the entanglement network of such a system will continue to complexify. As such, the quantum complexity of the system increases over time and this process far exceeds the time in which a closed system may reach equilibrium (maximal entropy state). This has important implications for black hole physics, and hence holographic and unified physics.

The Fallacy of Progress

Conventional evolutionary theory in biology is highly adverse to any proposition of an “intrinsic” tendency to increase in complexity. There are myriad examples of complex dynamical systems, however conventional theory holds that the increase in complexity is neither universal nor inevitable; Smith and Szathmary gave the example of bacteria as exemplifying this inference because many prokaryotes are probably no more complex today than their ancestors 2000 million years ago [20]. They maintain that the most we can say, in terms of the living organism, is that some lineages have become more complex in the course of time. As well, there are probably examples outside of biology of complex dynamical systems that have not exhibited a quantitative increase in their respective level of complexity, or information content, after reaching a dynamic equilibrium maximum. Smith and Szathmary further expounded key reasons why biologists tend to view any theory of an inevitable increase in complexity—amounting to a law of nature—as an erroneous inference and a fallacy, the fallacy of progress:

The notion of progress has a bad name among evolutionary biologists. Lamarck accepted the earlier idea of a ladder of nature and argued that organisms have an inherent tendency to climb the ladder. It was Lamarck’s notion of an inherent tendency, rather than his belief in the inheritance of acquired characters, that Darwin was rejecting when he said that his theory had nothing in common with Lamarck’s: he rightly saw that to explain evolution by an inherent tendency is as vacuous as to say that a man is fat because he has an inherent tendency to obesity. Today, we are unhappy with a picture of evolution that places us at the summit, and arranges all other organisms in a line behind us: what have we to be so proud about? To be fair, humans were by no means at the summit of the medieval scala naturae; there were angels and archangels above us as well as worms below.
There are, of course, more solid reasons, both empirical and theoretical, for rejecting a simple image of progress on a linear scale. Empirically, the history of life is better visualized as a branching tree than as a single ascending line. The fossil record shows that many organisms—horseshoe crabs, the coelacanth, crocodiles, for example—have undergone little change, progressive or otherwise, for hundreds of millions of years. On a shorter timescale, sibling species tell the same story. The fruit flies Drosophila melanogaster and D. simulans are hard to distinguish morphologically, but molecular data indicate that they are separated by several million years of evolution. Hence, either morphological evolution in the two species has been almost exactly parallel, which is implausible, or neither species has changed.
On the theoretical side, there is no reason why evolution by natural selection should lead to an increase in complexity, if that is what we mean by progress. At most, the theory suggests that organisms should get better, or at least no worse, at doing what they are doing right now. But an increase in immediate ‘fitness’—that is, expected number of offspring—may be achieved by losing eyes or legs as well as by gaining them. Even if an increase in fitness cannot be equated with an increase in complexity, or with progress, it might seem at first that R.A Fisher’s (1930) ‘fundamental theorem of natural selection’ at least guarantees an increase in fitness. The theorem states that the rate of increase in the mean fitness of a population is equal to the genetic variance in fitness: since variances cannot be negative, the theorem states that fitness can only increase. If so, ‘mean fitness’ in biology is an analogue of entropy in physics: it gives an arrow to time. Thus in physics the inevitable increase in entropy distinguishes past from future: if mean fitness can only increase, this defines a direction to evolution. It seems that Fisher did indeed think that his theorem could play such a role: otherwise, why ‘fundamental’? Unfortunately, the theorem holds only if the relative fitnesses of genotypes are constant, and independent of their frequencies in the population: for many traits, such constancy does not hold [ibid, 20].

So, while there may be a tendency—verging on a veritable physical law—for integrally-interacting multi-constituent dynamical systems to increase in complexity, it does not mean that every instance of such a dynamical system will necessarily increase in complexity, hence it is not absolutely necessary or universal. Some complex dynamical systems may reach an optimal information content early in their constitution, and therefore will remain relatively unchanging in an information optimum.

Unified Science- in Perspective

In the study The Autodidactic Universe [21] it is investigated whether it is possible that physical laws themselves can evolve and change. This is an interesting inquiry as it enables an evaluation of the reason why the present laws and constants of the universe are more likely than another set (known as the fine-tuning problem, which we also further discuss and clarify in our study The Unified Spacememory Network). So, for example, the coupling constants of nature (e.g., the gravitational constant G, or the fine-structure constant alpha) might turn out to be dynamical variables, and indeed in the Origin of Mass and Nature of Gravity [22] it is shown that fundamental properties like mass, the nuclear confinement or binding forces, and gravity are based on dynamical variables that are set by the conditions of decoherence of quantum vacuum fluctuations coupled with screening zero-point energy density and a resulting Planck pressure force that arises from the Planck plasma flow in black hole particles. The feedback dynamics operable in these fundamental states are quintessential information flows that characterize the organized functional change of evolving systems in the universe.

So, certainly there are mechanism underlying the complexification of our universe, and even the forces, constants, and laws themselves, which is a great thing because it means that we can come to understand these fundamental mechanisms and hence understand the nature of the universe at a deeper level. From the perspective of the Unified Spacememory Network, in which time (the 4^th dimension) is holographically emergent from the memory property of 3D voxelized space—i.e., time is not fundamental—it opens an interesting consideration on just what is meant by “evolving system” if all spacetime coordinates are co-existing simultaneously. What we point out in the Spacememory Network study is that the inherently atemporal nature of the universe and its inherent nonlocal nature (as readily exemplified in quantum theory) means that there is continual crosstalk in “evolving” systems between their initial state and much later states of high functional complexity, and hence there is a trans-temporal information exchange that is the negentropic ordering force in evolution and development of physical systems. The evolutionary trajectory of a given system is just the holographic projection of its underlying neuromorphic information connectivity network, i.e., the morphogenic field and ultimately change, like time, is illusionary.

References

[1] E. J. Chaisson, “A unifying concept for astrobiology,” International Journal of Astrobiology, vol. 2, no. 2, pp. 91–101, Apr. 2003, doi: 10.1017/S1473550403001484.

[2] A. Mistriotis, “A universal model describing the structure and functions of living systems,” Communicative & Integrative Biology, vol. 14, no. 1, pp. 27–36, Jan. 2021, doi: 10.1080/19420889.2021.1887549

[3] M. L. Wong et al., “On the roles of function and selection in evolving systems,” Proc. Natl. Acad. Sci. U.S.A., vol. 120, no. 43, p. e2310223120, Oct. 2023, doi: 10.1073/pnas.2310223120.

[4] T. Westby and C. J. Conselice, “The Astrobiological Copernican Weak and Strong Limits for Intelligent Life,” ApJ, vol. 896, no. 1, p. 58, Jun. 2020, doi: 10.3847/1538-4357/ab8225.

[5] S. A. Kauffman, The Origins of Order: Self-organization and Selection in Evolution. in The Origins of Order: Self-organization and Selection in Evolution. Oxford University Press, 1993. [Online]. Available: https://books.google.com/books?id=lZcSpRJz0dgC

[6] A. J. Watson, “Implications of an Anthropic Model of Evolution for Emergence of Complex Life and Intelligence,” Astrobiology, vol. 8, no. 1, pp. 175–185, Feb. 2008, doi: 10.1089/ast.2006.0115.

[7] Meijer D K F, Wong KW, 2022. How the Universe Orchestrated the Conditions for First Life, using an Informational Quantum Code. The Concerted Action of Magnetic Monopole and Photon/Phonon Fields through a 5D Symmetry Breaking. https://www.researchgate.net/publication/357312383_How_the_Universe_Orchestrated_the_Conditions_for_First_Life_using_an_Informational_Quantum_Code_The_Concerted_Action_of_Magnetic_Monopole_and_PhotonPhonon_Fields_through_a_5D_Symmetry_Breaking

[8] Sheldrake, R. (2009). Morphic Resonance: The Nature of Formative Causation. Ukraine: Inner Traditions/Bear.

[9] M. C. Parker and C. Jeynes, “Maximum Entropy (Most Likely) Double Helical and Double Logarithmic Spiral Trajectories in Space-Time,” Sci Rep, vol. 9, no. 1, p. 10779, Dec. 2019, doi: 10.1038/s41598-019-46765-w.

[10] N. Haramein, W. D. Brown, and A. Val Baker, “The Unified Spacememory Network: from Cosmogenesis to Consciousness,” Neuroquantology, vol. 14, no. 4, Jun. 2016, doi: 10.14704/nq.2016.14.4.961.

[11] W. Brown, “Provisional Definition of the Living State: Delineation of an Empirical Criterion that Defines a System as Alive,” Qeios online journal, preprint, Jul. 2023. doi: 10.32388/V5EDGF.

[12] A. Mistriotis, “Mathematical and physical considerations indicating that the cell genome is a read-write memory,” Progress in Biophysics and Molecular Biology, vol. 178, pp. 50–56, Mar. 2023, doi: 10.1016/j.pbiomolbio.2023.01.006.

[13] A. Wagner, Arrival of the Fittest, New York City: Penguin Group, 2014.

[14] M. Levin, “The Computational Boundary of a ‘Self’: Developmental Bioelectricity Drives Multicellularity and Scale-Free Cognition,” Front. Psychol., vol. 10, p. 2688, Dec. 2019, doi: 10.3389/fpsyg.2019.02688.

[15] R. M. Hazen, P. L. Griffin, J. M. Carothers, and J. W. Szostak, “Functional information and the emergence of biocomplexity,” Proc. Natl. Acad. Sci. U.S.A., vol. 104, no. suppl_1, pp. 8574–8581, May 2007, doi: 10.1073/pnas.0701744104.

[16] Mistriotis A. Self-similarity in living systems. Amazon. com Inc.; 2018 [accessed on November 11, 2023]. Kindle e-book: https://www.amazon.com/Self- similarity-Living-Systems-Antonis-Mistriotis-ebook /dp/B07G2DVMDL

[17] D. W. McShea, Functional complexity in organisms: Parts as proxies. Biol. Philos. 15, 641–668 (2000).

[18] Daniel W. McShea and Robert N. Brandon, Biology’s First Law: The Tendency for Diversity and Complexity to Increase in Evolutionary Systems. Chicago and London: The University of Chicago Press, 2010.

[19] A. R. Brown and L. Susskind, “The Second Law of Quantum Complexity,” Phys. Rev. D, vol. 97, no. 8, p. 086015, Apr. 2018, doi: 10.1103/PhysRevD.97.086015.

[20] J. M. Smith and E. Szathmary, The Major Transitions in Evolution. OUP Oxford, 1997

[21] S. Alexander et al., “The Autodidactic Universe,” arXiv:2104.03902 [gr-qc, physics:hep-th, physics:physics, physics:quant-ph], Mar. 2021, Accessed: Apr. 16, 2021. [Online]. Available: http://arxiv.org/abs/2104.03902

[22] Nassim Haramein, Cyprien Guermonprez, & Olivier Alirol. (2023). The Origin of Mass and the Nature of Gravity. https://doi.org/10.5281/zenodo.8381114